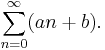Infinite arithmetic series
In mathematics, an infinite arithmetic series is an infinite series whose terms are in an arithmetic progression. Examples are 1 + 1 + 1 + 1 + · · · and 1 + 2 + 3 + 4 + · · ·. The general form for an infinite arithmetic series is
If a = b = 0, then the sum of the series is 0. If either a or b is nonzero, then the series diverges and has no sum in the usual sense.
Zeta regularization
The zeta-regularized sum of an arithmetic series of the right form is a value of the associated Hurwitz zeta function,
Although zeta regularization sums 1 + 1 + 1 + 1 + · · · to ζR(0) = −1⁄2 and 1 + 2 + 3 + 4 + · · · to ζR(−1) = −1⁄12, where ζ is the Riemann zeta function, the above form is not in general equal to
References
- Brevik, I. and H. B. Nielsen (February 1990). "Casimir energy for a piecewise uniform string". Physical Review D 41 (4): 1185–1192. doi:10.1103/PhysRevD.41.1185.
- Elizalde, E. (May 1994). "Zeta-function regularization is uniquely defined and well". Journal of Physics A: Mathematical and General 27 (9): L299–L304. doi:10.1088/0305-4470/27/9/010. (arXiv preprint)
- Li, Xinzhou; Xin Shi; and Jianzu Zhang (July 1991). "Generalized Riemann ζ-function regularization and Casimir energy for a piecewise uniform string". Physical Review D 44 (2): 560–562. doi:10.1103/PhysRevD.44.560.